In the last piece, I talked about my experience of emergence at the Emerge Gathering in Austin.
In this one, I’m going to talk about the geometry of group coherence.
We’re going to start fairly straightforward and then go a bit esoteric.
Human Geometry and Emergence
The simplest definition of emergence is when a complex system develops properties that are more than the sum of its parts.
When speaking of human groups, one good example of this is the phenomenon of collective intelligence. Let’s say you have 5 people talking together in a circle. If they are able to get into coherence with each other, a sixth entity can emerge - the bigger mind of the group as a whole. This is not the same as groupthink, or a situation where people in a group think the same way because of being exposed to the same ideology. This is an instance where people retain their individual perspectives, but are able to synergize them so that they are operating as a single unit, almost as if the individuals are different organs that are part of the same collective body.
At this point, people’s experience of reality may begin to synchronize and they may begin to understand each other implicitly, finishing each other’s sentences, or predicting what others are going to say before they say it. There is another thing that I have often observed in these situations - people tend to become a lot more cooperative. Instead of waiting for their turn to speak, they become in service to what wants to be said in the conversation, and are as happy to hear someone else say it as they are to say it themselves.
And this doesn’t just have to do with talking - small groups of people can synchronize with each other when it comes to doing all sorts of tasks. There is a very good reason why our ancestors did things in groups - hunting, foraging, child rearing, barn raising, dancing, and doing rituals. Not only did they get to socialize and take care of each other, but they also tapped into collective intelligence and collective sapience, which enabled them to do things they couldn’t do individually.
The way that this works is different depending on the number of people present. The kind of coherence that is possible between 5 people is different than that of 13 or 34.
How these numbers influence the kinds of coherence you can get in a group is something I have started referring to as human geometry. I don’t know if anyone else calls it that, it’s just something that made sense in my head. So human geometry it is.
The Question of Numbers
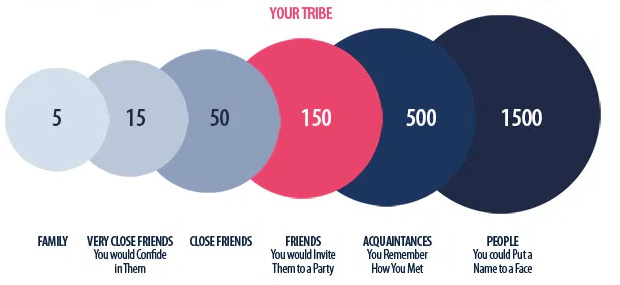
For hundreds of thousands of years, humans lived in small groups, and our brains evolved to be optimized for that context. British anthropologist Robin Dunbar famously popularized the idea that humans have a cognitive limit on how many meaningful relationships we can maintain, which is around 150 people. The image below indicates the different numbers of people we can maintain certain kinds of relationships with - from closest kin on the left to most distant acquaintances on the right. At the upper limit of around 1500, this is approximately how many people’s faces and names we can remember.
These numbers do vary somewhat from person to person, although there has been a lot of cross-cultural research and evidence that supports them. At the very least, they are useful to illustrate the general principle of how our social brains work. Research suggests that the above numbers are actually rounded for ease of remembering.
I suspect that the actual numbers are closer to those in the Fibonacci sequence, since that appears to be the math behind the organizing principle of many systems in our Universe.
The following is the Fibonacci sequence:
0,1,1,2,3,5,8,13,21,34,55,89,144,233,377,610,987,1597,2584, …
So the above diagram of Dunbar numbers may be more accurate with the numbers 5, 13, 55, 144, 610, and 1597 in place of the ones shown. Again, these numbers are approximate rather than exact.
If you are bothered by that, you may think of the phenomena as being wave-like or cluster-like rather than discreet. When you approach the apex of a wave, coherence gets easier, but it won’t be broken if you are off by a few numbers. This kind of flexibility is adaptive in living systems.
There’s a “there” there, even if the numbers are just the proverbial finger pointing at the moon. We can think of them like ripples in the pond that suggest that something has fallen into the water even though we didn’t see the thing that fell.
There is a higher order to how life organizes itself, even if we don’t fully understand it.
Collective Intelligence and Coherence in Groups
Understanding Dunbar and Fibonacci numbers is useful when we want to create groups to support different forms of coherence. I’ll name as few examples from my experience below.
If we want to facilitate deep conversation that creates intimacy and trust, 5 people may be the right size of group.
If we are dealing with solving complex societal or systemic issues, a different group size may be appropriate. In my work of facilitating collective intelligence practice, I found that groups around 5-13 people are optimal for that task. It’s a lot harder to maintain the same quality of conversation past that number. The Council of 13 Indigenous Grandmothers was no accident.
If we want to help people nurture friendships and family ties, groups of around 34-55 may be a good size. For example, my wedding had around 34 people and was just right.
And if we want to generate a sense of community and work on a big project together, we can aim to have a group between 55 and 144. This is where we get to the size of an ancestral village.
For example, Amish barn raising can involve 50-150 people, and a particular Bulgarian bagpipes festival famously features over 100 pipers playing simultaneously (yes, it’s deafening and magical).
You get the idea of what sorts of group sizes are suitable for what purposes.
Human geometry plays an important role in facilitation, be it at conferences, retreats, festivals, informal gatherings, or other types of events. It can allow us to be intentional when we create spaces that are meant to enable emergence. Of course, there is a lot more to emergence than geometry, but it was worth talking about it as the structural element.
In the next piece, I will talk about synchronization and energetics in groups.







#teamci
CI is da thing!